Should we teach students math shortcuts and tricks?

Shortcuts are a necessary part of math. If you are an efficient problem solver, you are likely using shortcuts that you have learned. We want students to use formulas, and formulas are shortcuts. Many procedures and algorithms are shortcuts that save time, allowing us to go deeper and deeper in the math (hopefully).

The approach to shortcuts is what we must examine.

Are we showing students all the shortcuts without allowing time for them to understand why they work?

If we are showing students shortcuts, students are likely memorizing them in order to get an answer that day or pass a quiz that week. Memorization without understanding causes problems later. Without the conceptual understanding of what is happening, students forget. They apply the shortcuts at the wrong time, and they cannot extend them to other concepts. And it sends the message that math makes no sense, which may be the most worrisome outcome of all.

Are students figuring out their own shortcuts?

If students are finding patterns and efficient methods on their own, they are generalizing, which is an important math skill. When they make sense of the math, they are more likely to remember how the shortcut works and why it works. When they understand why and how, they can apply the same logic to more and more complex problems. Plus, their confidence in math grows. Allowing students to figure something out is one of the best ways for students to see themselves as “math people.”

What are the most misused math shortcuts and tricks?

I asked teachers on Instagram to tell me what shortcuts they see their students using incorrectly. Many times students learn a shortcut in school or at home in order to get an answer, but they go on to apply that shortcut incorrectly later. Without understanding the math, the shortcut ends up leaving the student with misconceptions and confusion.

Below are some of the most commonly misused shortcuts, plus some recommendations for different approaches.

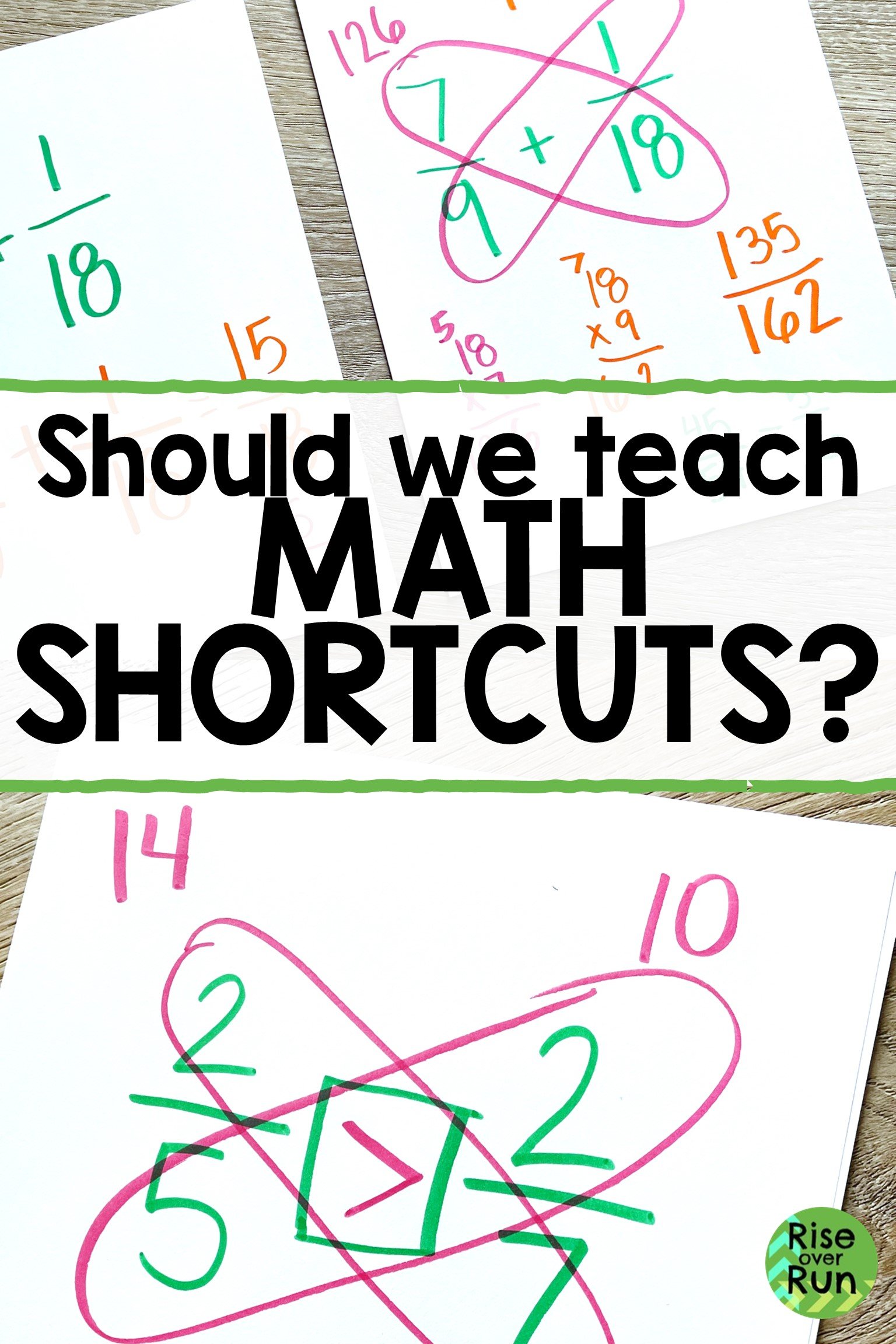
Butterfly Method

The “butterfly method” is often used for comparing fractions, adding fractions, or subtracting fractions. Students draw a butterfly figure around 2 fractions and multiply each numerator by the other fraction’s denominator.

Although the butterfly procedure may work for getting an answer, it has 2 big issues. Students usually have no idea why it works, and they often use it at incorrect times. (I’ve taught plenty of middle schoolers who try to use a butterfly to multiply fractions.)

What to do instead:

When comparing fractions, students need a firm understanding of what a fraction represents. If a student is not ready to compare 2/5 and 2/7 mentally, they should not be given a shortcut to the answer. Instead, manipulatives and visuals are vital to building that conceptual understanding. Learning where benchmark fractions fall on a number line will help students compare as well.

Comparing fractions may not seem like an important skill, but it is an important indicator as to whether or not students understand what a fraction means. If they don’t, they’ll have a difficult time working with fractions in other applications.

When adding and subtracting fractions, avoid the butterfly as well. The butterfly method is built off the idea of creating common denominators, but it isn’t always the most efficient. For example, to add 7/9 and 1/18, 18 can be used as a common denominator. With the butterfly method, many students would create more work for themselves. We want students to be efficient problem solvers, not thoughtless calculators!

Keep Change Flip

“Keep, change, flip” is a shortcut used for dividing fractions. If students are taught this shortcut when they are first introduced to dividing fractions, they likely will not have the opportunity to think deeply about fraction division.

What to do instead:

Fraction division… it is a tough concept to think about, even for many adults. However, just because a concept is different or challenging does not mean it is impossible.

Manipulatives, visuals, and context are critical for making fraction division make sense. Around 5th grade, students start dividing whole numbers by unit fractions and dividing unit fractions by whole numbers with problems like 5 ÷ 1/3 or 1/3 ÷ 5. These types of problems are important for students to explore visually before being told a procedure. If they are allowed time to think and try, they can pick up on the patterns themselves.

As students move into 6th grade, they usually get into more complicated fraction division problems. These may be more challenging, but exploration with visuals like tape diagrams will help build the conceptual understanding. If students are using procedures to get an answer, they need to be able to explain why it works.

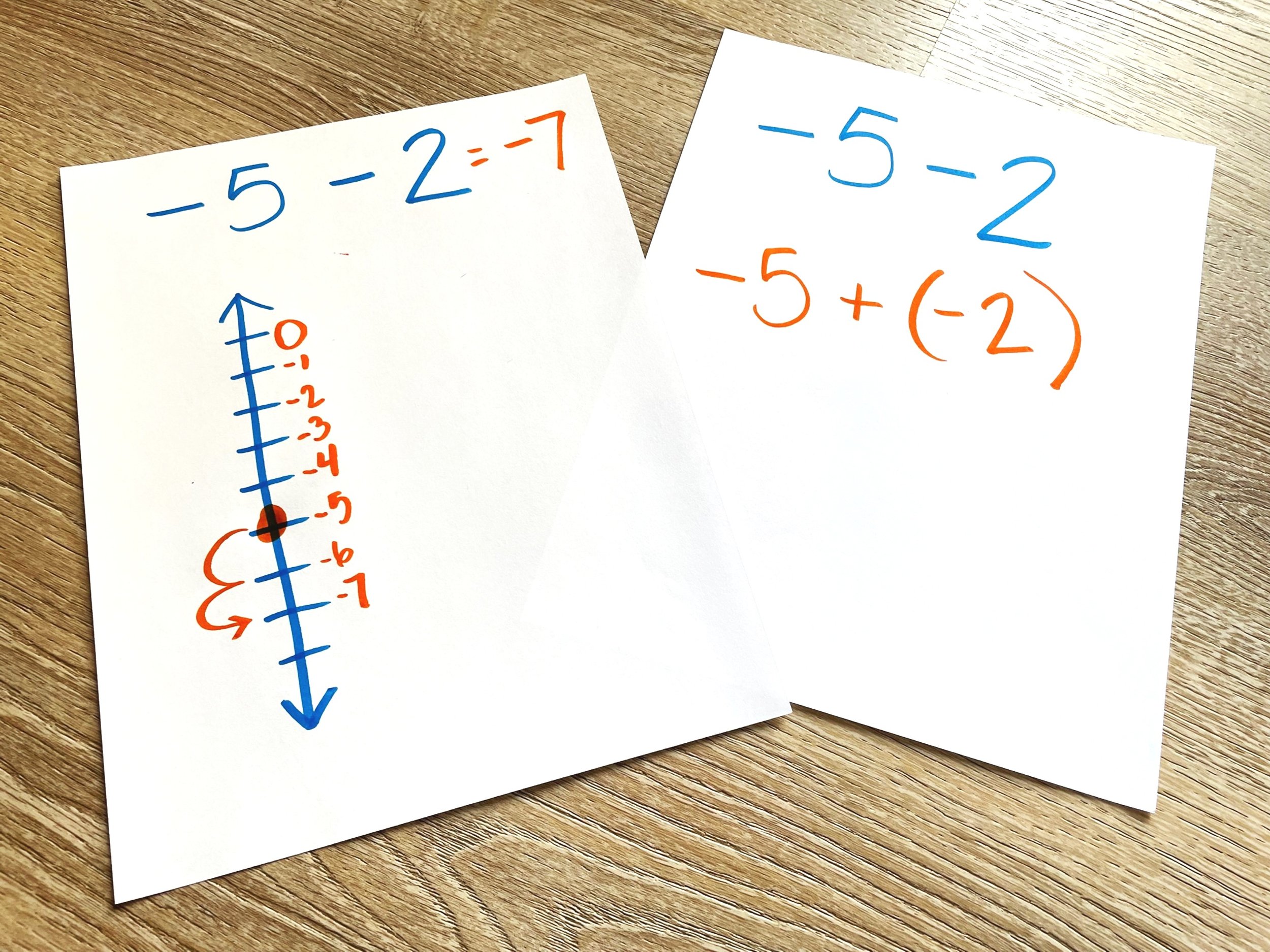
Keep Change Change

“Keep, change, change” is a shortcut that students sometimes learn in order to make an integer subtraction problem into an addition problem. For example, using “keep, change, change,” students would rewrite -5 - 2 as -5 + (-2).

It is important for students to understand that the 2 expressions above are equivalent. Changing the expression isn’t necessarily an issue if it helps them think about the math. The problem is when students learn this shortcut as an alternative to developing a deep understanding of integers.

What to do instead:

Although students encounter negative numbers in the real world, it can be intimidating to start learning integer operations. It’s new. First, students need to have an understanding of what negative numbers represent. A number line and real world context help a lot. Then students need to be able to compare negative values. Once those are mastered, students can start thinking about adding and subtracting integers.

Avoid giving students integer operation rules or tricks like “keep change change” in the beginning. Instead, let them think about problems with real world context using manipulatives or number lines. For example, if a submarine is 5 km below sea level and descends 2 km, what is its depth? Letting them explore and notice patterns will develop their conceptual understanding.

What can we do if students already know the shortcuts, but don’t understand the math?

It’s always tough when students have learned a shortcut to an answer but have no clue why it works. As math teachers who want to see our students truly understand the “why” behind the steps, we can do a few things when this happens.

Emphasize that the answer is not as important as the understanding. (An answer only helps you today, understanding helps you in the future.)

We can phrase questions in ways that ask students to explain what is happening. Word problems and tasks that get students problem solving often require more thought than a shortcut.

Ask students to analyze different solution methods. Show different methods, even shortcuts, and ask students to try to explain if they agree or disagree with the work.

Ask for proof. Question students, “Will that always work?” Challenge them to really prove why their shortcut works and to know when they will be able to use it.

Shortcuts and Math Enthusiasm

A final thought on shortcuts…

It isn’t that shortcuts are inherently good or bad. It’s how we utilize shortcuts in math education.

So many people who dislike math view the subject as a bunch of meaningless rules. Being told lots shortcuts and procedures to follow (which often later get confused and used incorrectly) contributes to the feeling that math makes no sense.

On the other hand, figuring out a shortcut for yourself is very empowering in math. A student working with integers and realizing that adding a negative is the same as subtracting a positive will feel very proud. When a student sees (with visuals) that dividing 5 by 1/3 can be solved by multiplying by 3, they see that math makes sense.

The more opportunities we give students to discover their own shortcuts, the more opportunities they have to build math enthusiasm and understanding.

Here are some resources you might find helpful for some of the topics mentioned in this blog post.